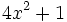Differenze tra le versioni di "Matematica discreta/Esami/2002-xx-xx"
| (2 versioni intermedie di 2 utenti non mostrate) | |||
| Riga 1: | Riga 1: | ||
Compitino prof. [[Bonzini]] del '''2002''' | Compitino prof. [[Bonzini]] del '''2002''' | ||
| + | |||
| + | #<math> \gamma : \begin{pmatrix} x \\ y \\ z \end{pmatrix} \Rightarrow \; \begin{pmatrix} x+2y-z\\ 2x+4y-2z \end{pmatrix}</math> (funzione da <math> \mathbb{R}^3 </math> a <math> \mathbb{R}^2 </math>) | ||
| + | ## '''<math> \gamma </math>''' è omomorfismo di spazi vettoriali | ||
| + | ## <math> \gamma ( \mathbb{R}^3 ) </math> è la retta di <math> \mathbb{R}^2 </math> generata da <math> {1 \choose 2} </math> | ||
| + | ## ogni classe della '''<math> \rho_\gamma </math>''' è un piano parallelo a <math> \langle \begin{pmatrix} 1 \\ 0 \\ 1 \end{pmatrix} , \begin{pmatrix} 0 \\ 1 \\ 2 \end{pmatrix} \rangle </math> | ||
| + | ## il piano <math> \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix} + \langle \begin{pmatrix} 1 \\ 0 \\ 1 \end{pmatrix} , \begin{pmatrix} 1 \\ 1 \\ 3 \end{pmatrix} \rangle </math> è una classe della relazione '''<math> \rho_\gamma </math>''' | ||
| + | #quoziente e resto in <math> (\mathbb{Z}_7 [x],+,\cdot) </math> di <math> x^4+3x+2 </math> con <math> 4x^2+1 </math> | ||
| + | |||
| + | |||
| + | <gallery> | ||
| + | Immagine:Compito-1.jpg|Compitino | ||
| + | </gallery> | ||
| + | |||
| + | == Soluzione == | ||
<gallery> | <gallery> | ||
| − | |||
Immagine:Compito-2.jpg|02 | Immagine:Compito-2.jpg|02 | ||
Immagine:Compito-3.jpg|03 | Immagine:Compito-3.jpg|03 | ||
Versione attuale delle 17:58, 7 gen 2008
Compitino prof. Bonzini del 2002
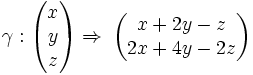 (funzione da
(funzione da 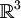 a
a  )
)
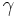 è omomorfismo di spazi vettoriali
è omomorfismo di spazi vettoriali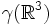 è la retta di
è la retta di  generata da
generata da 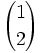
- ogni classe della
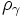 è un piano parallelo a
è un piano parallelo a 
- il piano
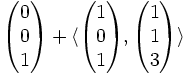 è una classe della relazione
è una classe della relazione 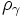
- quoziente e resto in
![({\mathbb {Z}}_{7}[x],+,\cdot )](/images/math/9/2/8/9282860f41f9dcb89143fcb348b5239f.png) di
di 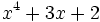 con
con